角分辨光电子能谱

EX7课题组以ARPES实验技术为主，兼具薄膜生长与STM/ARPES原位测量，并发展了多种原位调控手段，研究拓扑、关联、超导、低维等量子材料体系在动量空间和实空间的电子态。自2008年课题组成立以来，在铁基超导体、拓扑半金属、铁基拓扑超导等多个领域取得了突破性的研究进展，已发表1篇Reviews of Modern Physics、2篇Nature、3篇Science、6篇Nature Physics、9篇Physical Review X、22篇Physical Review Letters、13篇Nature Communications、4篇Science Advances。

在铁基超导体领域，在Ba_0.6K_0.4Fe₂As₂中观测到依赖于费米面的无节点超导能隙分布【EPL 83, 47001 (2008)】，被国际同行公认是对铁基超导体的s波配对对称性的建立具有奠基性意义的工作，并被Physics、EuroPhysics News、Science Watch、Physics Today等杂志作为铁基超导领域的突破性成果多次点评；在K_1-xFe_2-ySe₂超导体中发现布里渊区中心空穴型费米面消失【PRL 106, 187001 (2011)】，打破了早期流行的费米面嵌套诱导的反铁磁涨落导致铁基超导电子配对的观点，作为代表性论文之一获得2021年国家自然科学奖二等奖。

在拓扑半金属领域，与物理所、人大等单位的理论计算和材料制备团队合作，首次实验证实外尔费米子【PRX 5, 031013 (2015)、Nature Physics 11, 724 (2015)】、三重简并费米子【Nature 546, 627 (2017)、Nature Physics 14, 349 (2018)】、非传统手性费米子【Nature 567, 496 (2019)】，应邀在Reviews of Modern Physics【RMP 93, 025002 (2021)】等期刊上撰写综述。部分工作入选“物理评论”系列期刊125周年纪念论文集（共49项，其中34项获诺贝尔奖）、中科院改革开放四十年40项标志性重大科技成果—“拓扑物态领域系列研究”、英国物理学会《物理世界》评选的2015年度物理学十大突破、美国物理学会《物理》评选的2015年物理学八大亮点工作、2015和2017年中国十大科技进展新闻、2015和2017年中国科学十大进展。

在铁基拓扑超导领域，与物理所、东京大学等单位的多个理论和实验团队合作，提出铁基超导体中可能存在能带反转导致的拓扑Dirac表面态【PRB 92, 115119 (2015)】，首次在铁基超导体中观测到拓扑表面态及表面态上的s波超导能隙【Science 360, 182 (2018)】，在磁通涡旋中观测到马约拉纳零能模，即马约拉纳任意子【Science 362, 333 (2018)】，观测到磁通涡旋中能级的半整数位移，证实了其拓扑非平庸的本质【Nature Physics 15, 1181 (2019)】，观测到马约拉纳零能模的近量子化电导平台特征【Science 367, 189 (2020)】。部分工作入选中科院改革开放四十年40项标志性重大科技成果—“拓扑物态领域系列研究”和2018年中国十大科技进展新闻。

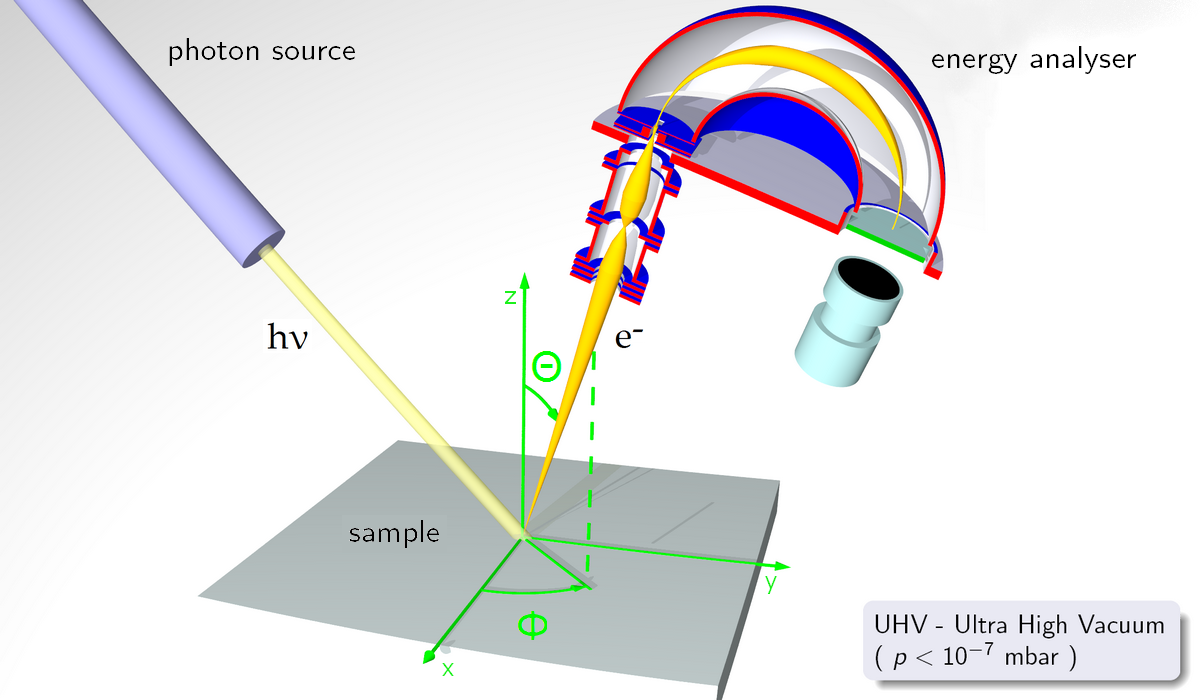
ARPES(角分辨光电子能谱)是利用1887年赫兹发现的光电效应研究固体中的电子结构。当一特定频率的光照射在表面上，一些接近表面的电子吸收入射光子能量激发到表面外的现象叫做光电效应。在ARPES实验中，我们使用惰性气体放电灯或同步辐射作为光源，并有电子分析器收集并分析出射的电子。如今，典型的电子收集器是测量出射光电子的动能以及角度信息。通过能量和动量守恒，我们可以得到样品内部的电子结构。如上图所示，ARPES技术可以直接测量材料中的电子结构，因此它是研究量子材料的有力工具。

ARPES (Angle Resolved PhotoElectron Spectroscopy) makes use of the photoelectric effect discovered by Heinrich Hertz in 1887 to study the electronic structure of solids. The photoelectric effect is demonstrated when light above a certain frequency shines on a surface causing some electrons near the surface to be ejected when they absorb energy from the incident photons. In our ARPES experiments, we typically use a noble gas discharge lamp or synchrotron radiation as the light source. An electron analyzer then collects some of the ejected electrons for analysis.

Typical analyzers in use today measure the number of electrons as a function of the emission angle (or equivalently, the momentum) and the kinetic energy of outgoing photoelectrons. By making use of the laws of conservation of energy and momentum, we can calculate what the momentum and binding energy values were when the electron was in the sample.

For the momentum, we usually can only determine the momentum of the electrons inside the solid along directions parallel to the sample surface. This is because that, while the momentum is conserved along the parallel directions, it is not conserved in the normal direction due to the symmetry breaking on the surface.

For details: ARPES-Wiki